Particle in a Box
We are going to discuss a single particle in a box classical as well as quantum. Classically: Particle confined in a box and walls of the box are completely rigid. So it can not penetrate the walls of the box. Particle have momenta p so it has kinetic energy E and it can undergo with elastic collision to the wall of the container. Elastic collision simply means that momenta and kinetic energy of the particle both are conserved. Particle can bounce back from the wall of the container and exert pressure to the wall by the momentum transfer.
Rigid wall some time treated as a infinite potential. It means particle is not free. It is completely in the bound state. The particle cannot penetrate the wall of the box. Quantum Mechanically: Quantum mechanically situation is completely different when particle confined in a box. Standing waves generates in the box and zero potential energy is not possible. If the wall of the container are not perfectly rigid means potential is finite then there is a certain probability that the particle penetrates the wall of the container and this phenomenon is known as quantum tunneling. Now we will understand how quantum mechanically particle behave in a box. According to the quantum mechanics every particle can be described by its state, which is known as quantum state or the wave function of the particle.

Quantum state is an abstract concept which can be defined mathematically in Hilbert space. But simply square of the wavefunction describe the probability density of the particle. As quantum mechanics says that we can not define exact position and momentum of the particle so there is always a probability of the particle in a certain state. We can solve Schrodinger equation to get the appropriate solution of the wave function. Main thing is that wave function should vanish at the boundary. There are infinitely many solutions possible for the particle and according to the one solution, there is one possibility to penetrate the wall by the particle.
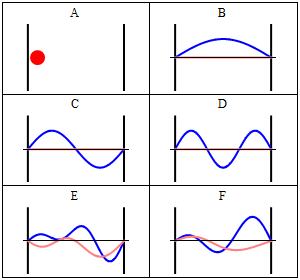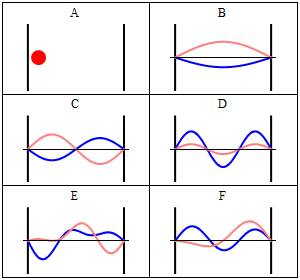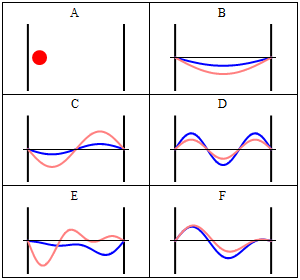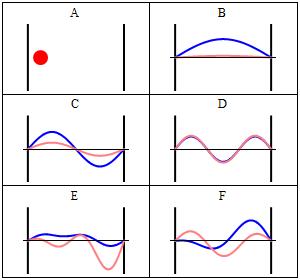
Here question arises, how it can be possible? It is a counter-intuitive example from daily life but there are certain things in nature where we can fit this. Decay of alpha particle from the nucleus. Alpha decay is a very well known phenomenon in the radioactivity. We know that energy of the alpha particle is very less compared to the energy of the nucleus but still it penetrates the nucleus. This phenomenon cannot be explained classically but quantum mechanically it can be explained.
